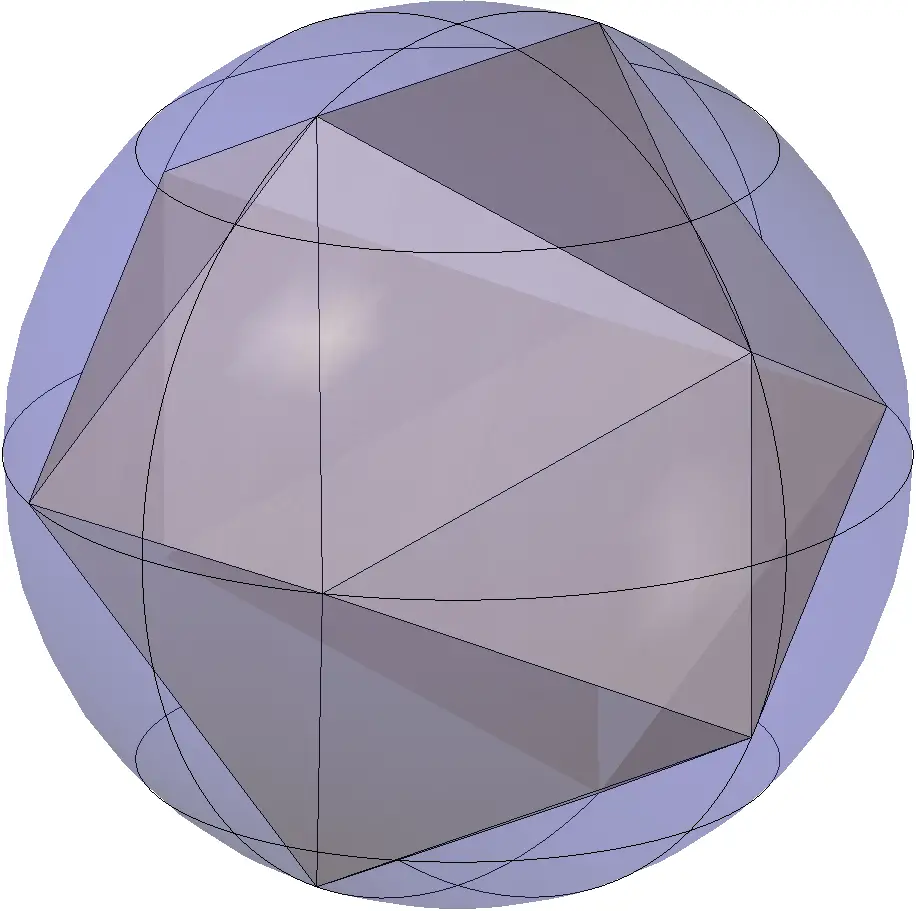
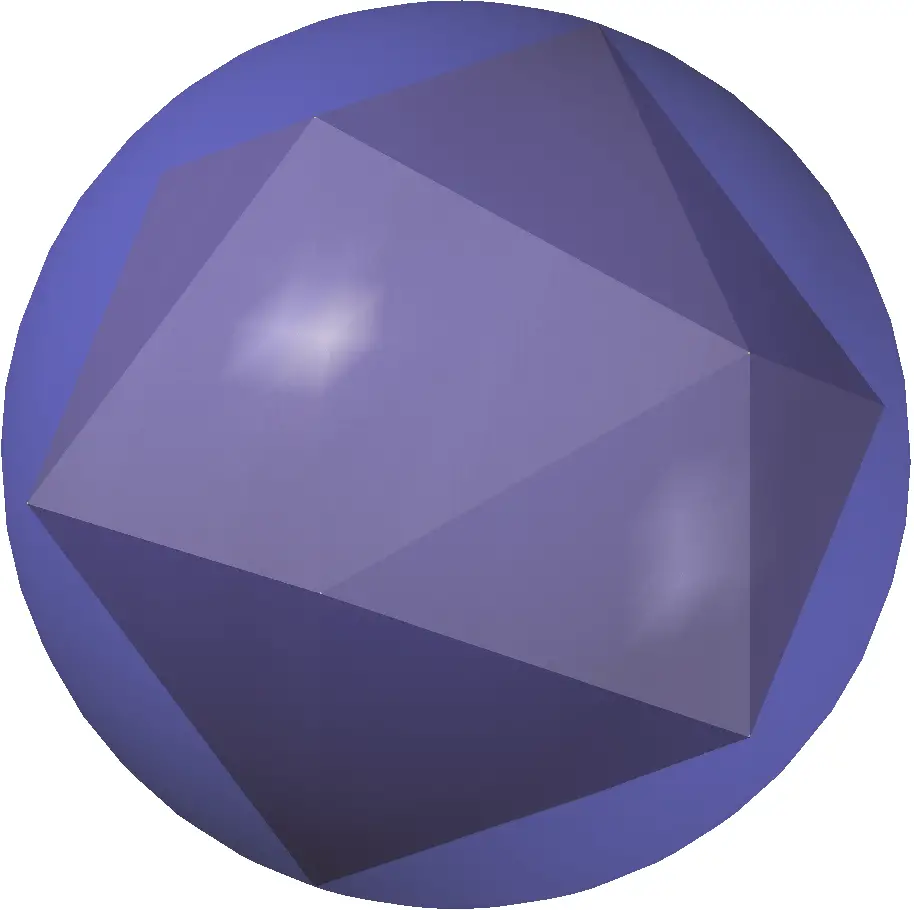
What is a polyhedron?
A polyhedron is a solid which is limited only by flat surfaces which we call faces, intersections of faces are called edges, and points where edges are cut are called corners. Diagonal lines are called lines that join corners that do not belong to the same face.
What is a regular polyhedron?
As you can imagine, a regular polyhedron is one that all its faces are regular polygons. In total, there are only 5 regular polyhedrons that you already know, each of these polyhedrons has the prefix of the number of faces. Let’s see the figure broken down as it would look without being armed and let’s see the armed figure:
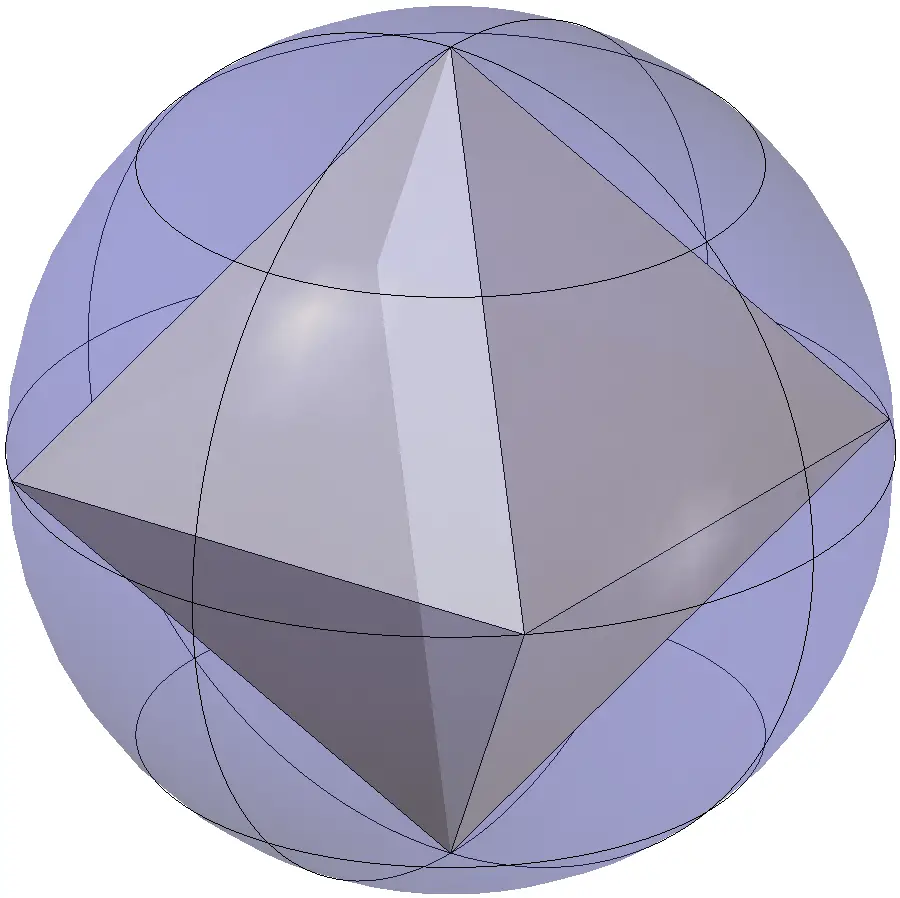
Regular tetrahedron
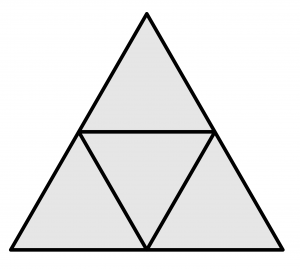

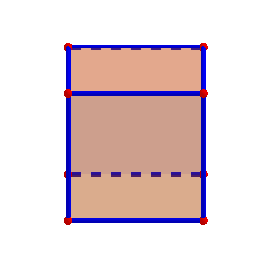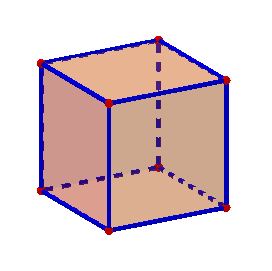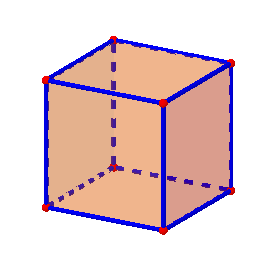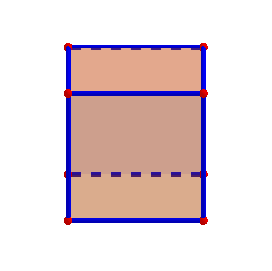
Regular Hexahedron or cube


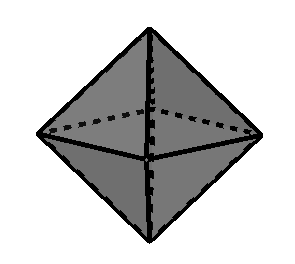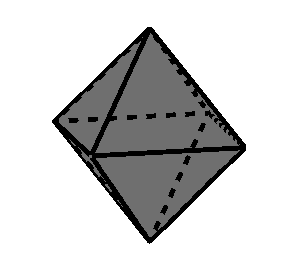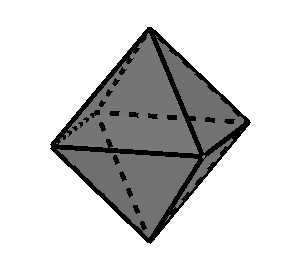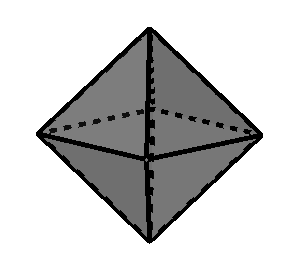
Regular octahedron
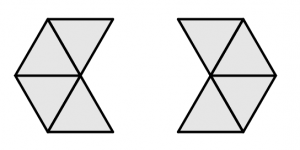

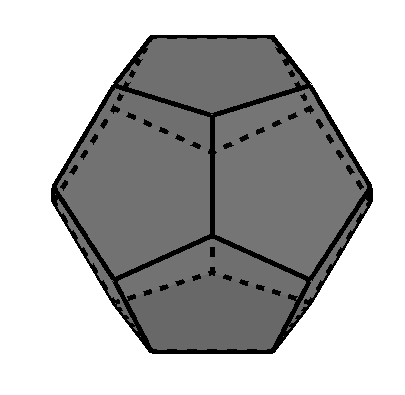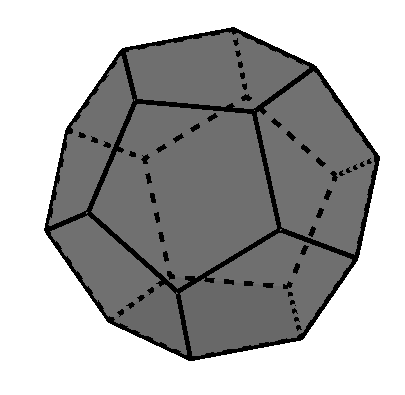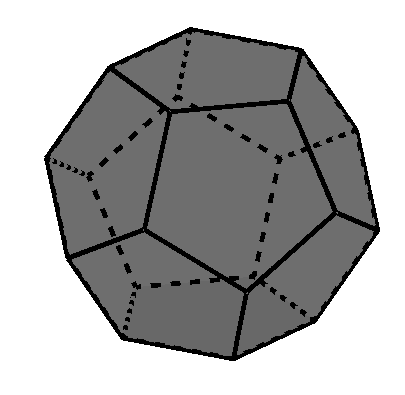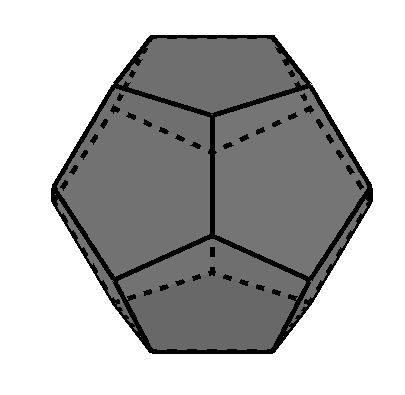
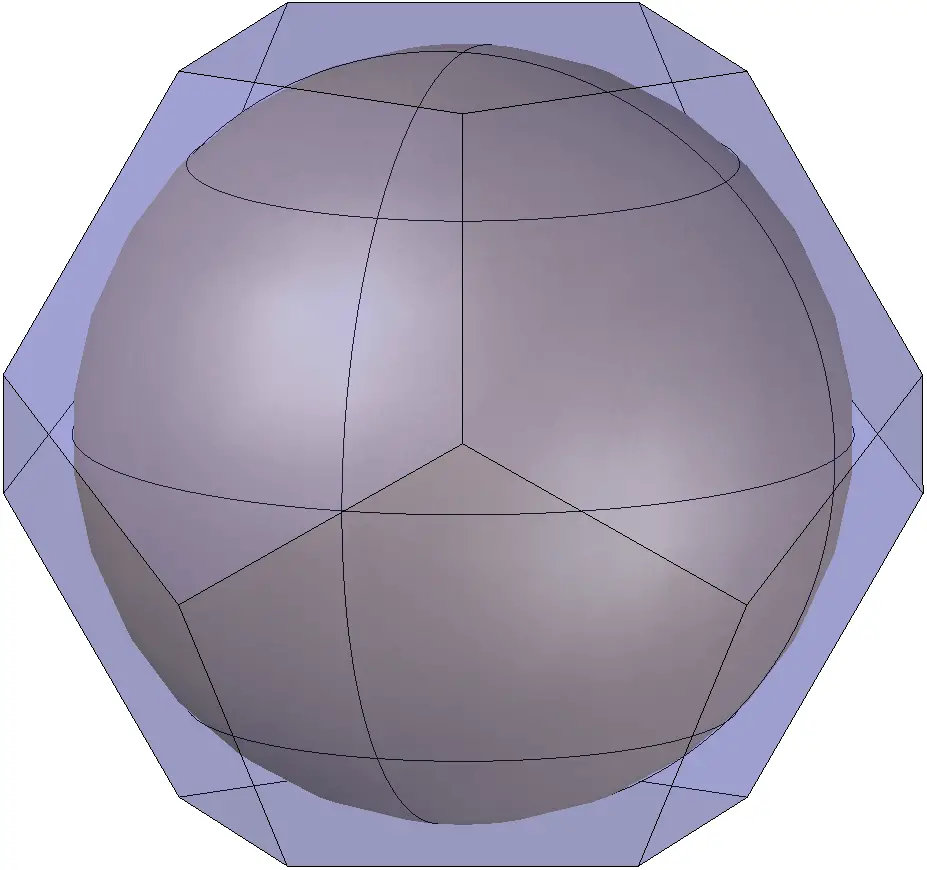
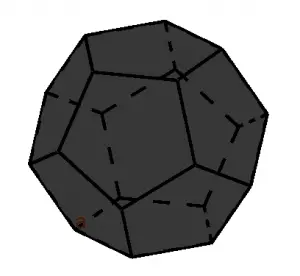
Regular dodecahedron
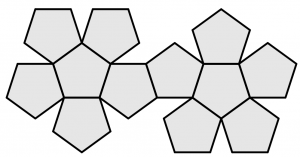
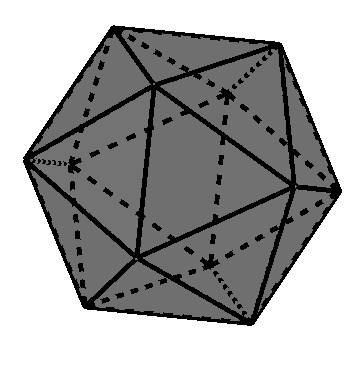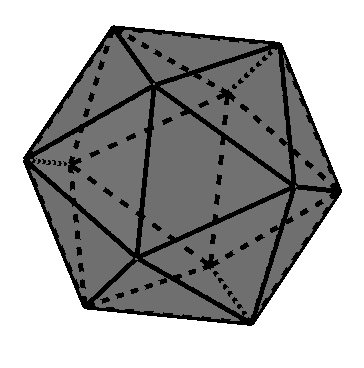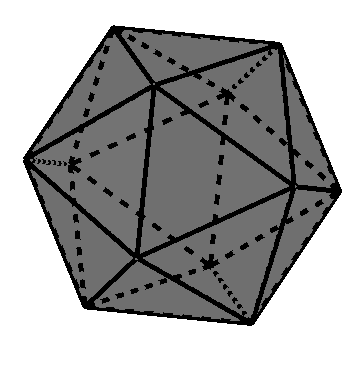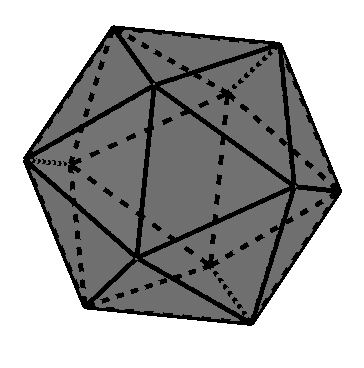
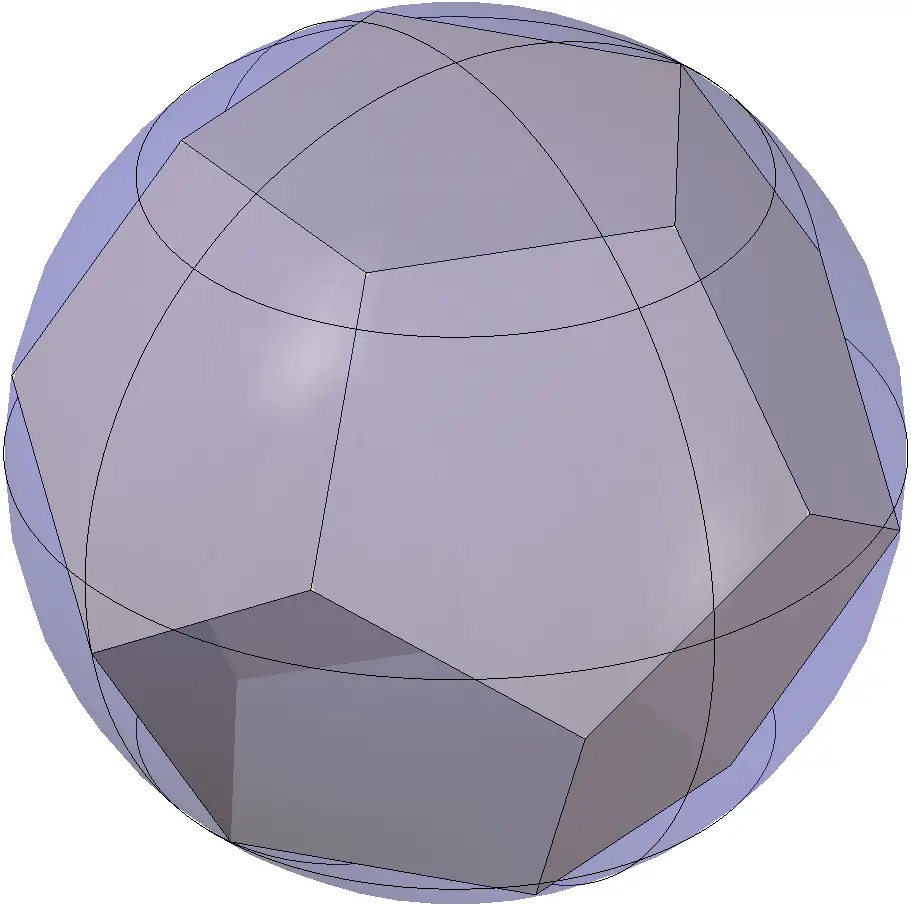
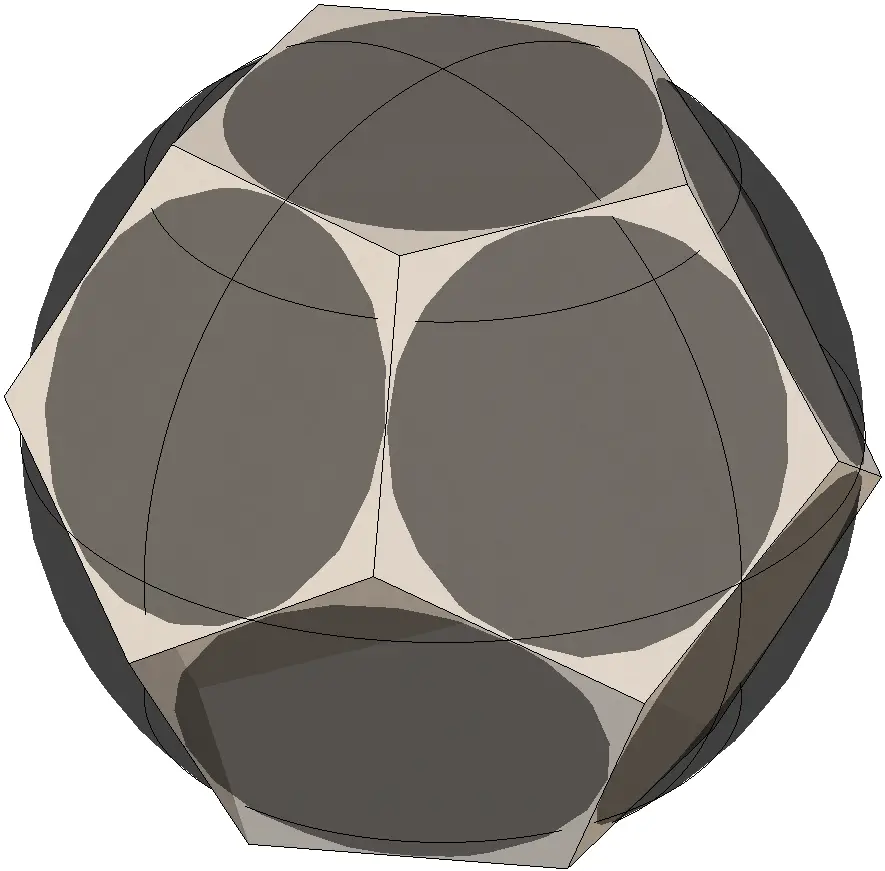
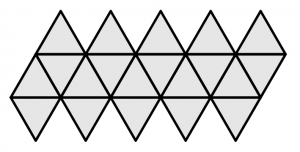
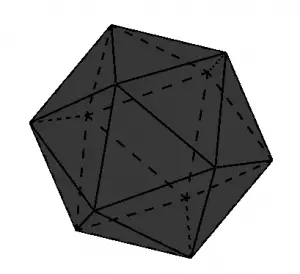
Icosahedron regular
Areas and volumes of regular polyhedrons
First we must take into account the following in order to calculate the area, volume and radius of the regular polyhedrons:
A = area
V = volume
a = edge
R = radius of the circumscribed sphere
r = radius of the inscribed sphere
\rho = radius of the sphere tangent to the edges
Formulas for the calculation of the area, volume and radios of a tetrahedron
Area of a tetrahedron
A = a^{2}\sqrt{3} = \cfrac{8}{3} \ R^{2}\sqrt{3} = 24r^{2} \sqrt{3} = 8 \rho \sqrt{3}
Volume of a tetrahedron
V = \cfrac{a^{3}}{12} \sqrt{2} = \cfrac{8}{27} \ R^{3}\sqrt{3} = 8r^{3} \sqrt{3} = \cfrac{8}{3} \ \rho^{3}
Radios of a tetrahedron
R = \cfrac{a}{4} \sqrt{6} , \quad r = \cfrac{a}{12}\sqrt{6}
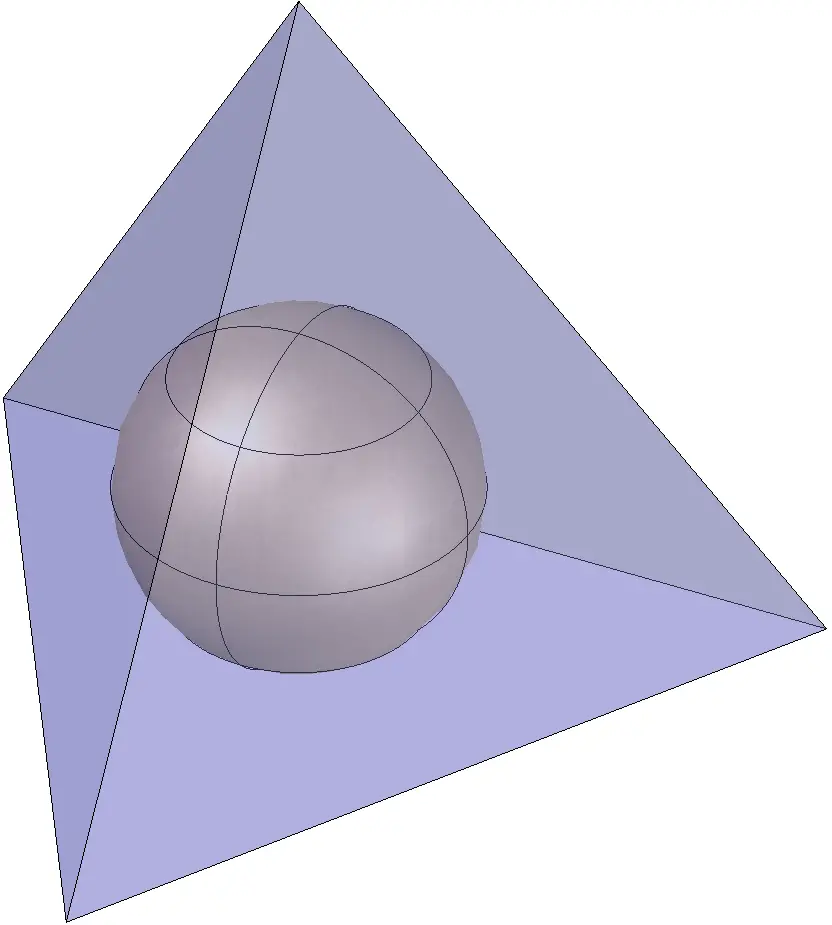
Tetrahedron circumscribed in the sphere
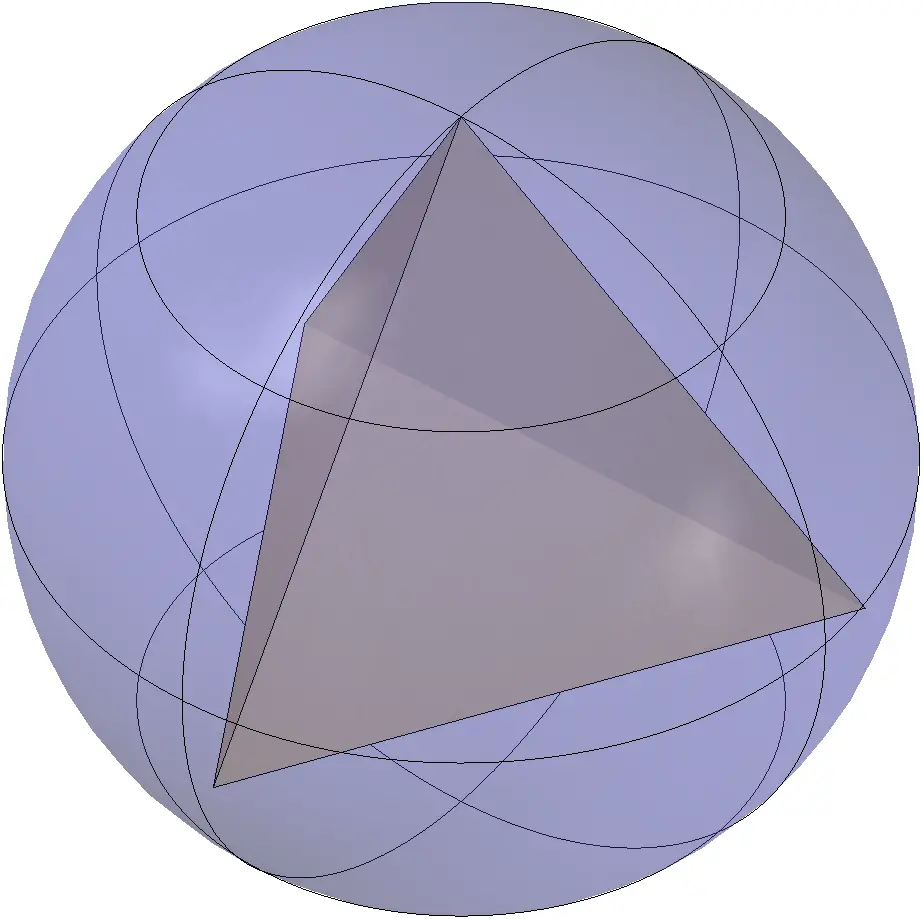
Tetrahedron inscribed in the sphere
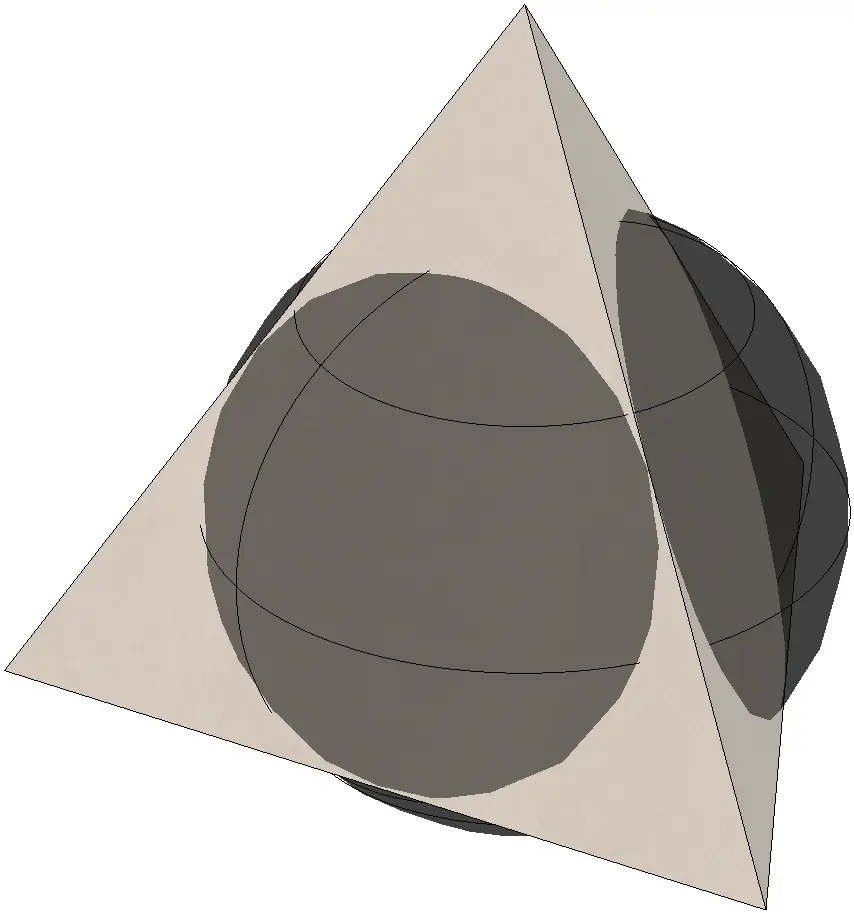
Tetrahedron tangent to the sphere
Formulas for calculating the area, volume and radios of a hexahedron or cube
Area of a cube
A = 6a^{2} = 8R^{2} = 24r^{2} = 12 \rho ^{2}
Volume of a cube
V = a^{3} = \cfrac{8}{9} \ R^{3}\sqrt{3} = 8r^{3} = 2\rho^{3}\sqrt{2}
Radios of a cube
R = \cfrac{a}{2}\sqrt{3}, \quad r = \cfrac{a}{2}
Cube circumscribed in the sphere
Cube inscribed in the sphere
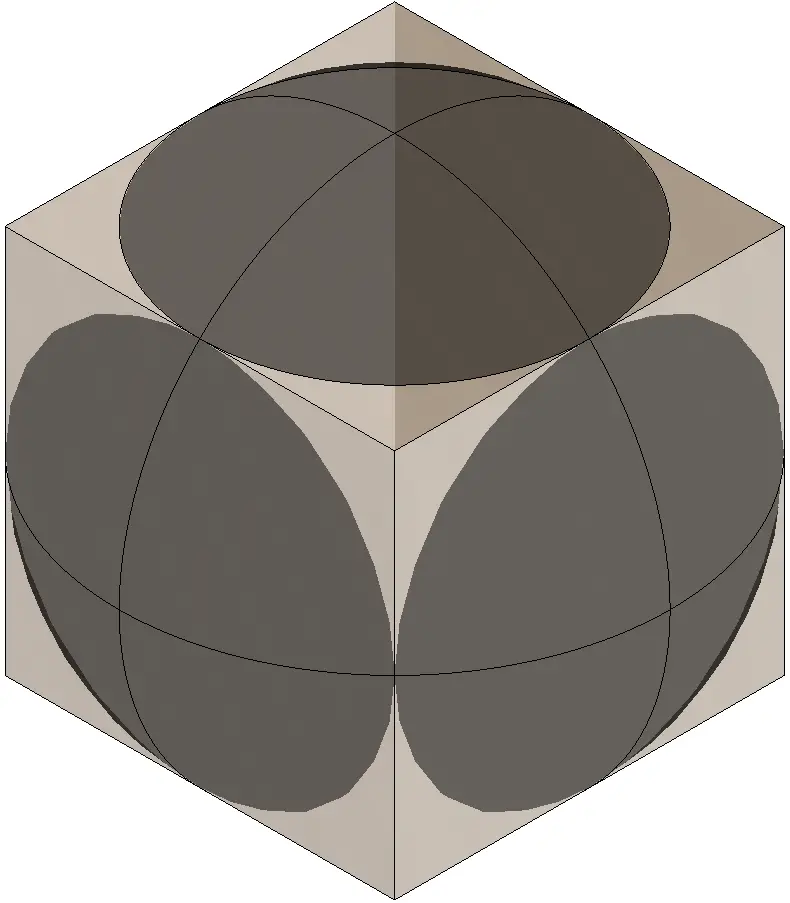
Cube tangent to the sphere
Formulas for the calculation of the area, volume and radios of an octahedron
Area of an octahedron
A = 2a^{3}\sqrt{3} = 4R^{2} \sqrt{3} = 12r^{2}\sqrt{3} = 8 \rho ^{2}\sqrt{3}
Volume of an octahedron
V = \cfrac{a^{3}}{3}\sqrt{2} = \cfrac{4}{3} \ R^{3} = 4 r^{3} \sqrt{3} = \cfrac{8}{3} \ \rho^{3} \sqrt{2}
Radios of an octahedron
R = \cfrac{a}{2}\sqrt{2}, \quad r = \cfrac{a}{6}\sqrt{6}
Octahedron circumscribed in the sphere
Octahedron inscribed in the sphere
Octahedron tangent to the sphere
Formula for the calculation of the area, volume and radios of a dodecahedron
Area of a dodecahedron
A = 3 a^{2}\sqrt{5 \left ( 5 + 2\sqrt{5} \right ) } = 2 R^{2} \sqrt{10 \left ( 5 - \sqrt{5} \right )}
A = 30r^{2} \sqrt{2\left ( 65 - 29 \sqrt{5} \right )} = 6\rho^{2}\sqrt{10 \left (25 - 11 \sqrt{5} \right )}
Volume of a dodecahedron
V = \cfrac{a^{3}}{4} \left ( 15 + 7\sqrt{5} \right ) = \cfrac{2 R^{3}}{9}\left ( 5\sqrt{3} + \sqrt{15} \right )
V = 10r^{3} \sqrt{2 \left ( 65 - 29\sqrt{5} \right )} = 2\rho^{3} \left ( 3\sqrt{5} - 5\right )
Radios of a dodecahedron
R = \cfrac{a}{4}\left ( \sqrt{3} + \sqrt{15} \right ), \quad r = \cfrac{a}{20}\sqrt{10 \left ( 25 + 11 \sqrt{5} \right )}
Dodecahedron circumscribed in the sphere
Dodecahedron inscribed in the sphere
Dodecahedron tangent to the sphere
Formulas for calculating the area, volume and radios of an icosahedron
Area of an icosahedron
A = 5a^{2} \sqrt{3} = 2R^{2}\sqrt{3} \left ( 5 - \sqrt{5} \right )
A = 30r^{2}\sqrt{3} \left ( 7 - 3\sqrt{5} \right ) = 10 \rho^{2}\sqrt{3}\left ( 3 - \sqrt{5} \right )
Volume of an icosahedron
V = \cfrac{5a^{3}}{12} \left ( 3 + \sqrt{5} \right ) = \cfrac{2R^{3}}{3} \left ( \sqrt{10 + 2\sqrt{5}}\right )
V = 10r^{3} \sqrt{3} \left ( 7 - 3 \sqrt{5} \right ) = \cfrac{10 \rho^{3}}{3}\left ( \sqrt{5} - 1 \right )
Radios of an icosahedron
R = \cfrac{a}{4} \sqrt{10 + 2 \sqrt{5}}, \quad r = \cfrac{a\sqrt{3}}{12} \left ( 3 + \sqrt{5} \right )
Icosahedron inscribed in the sphere
Icosahedron inscribed in the sphere, more realistic
The formulas mentioned above were taken from the following reference:
Spiegel, M. (1999). Manual de fórmulas y tablas matemáticas, D.F.,México, McGRAW-HILL.
Thank you for being at this moment with us :)