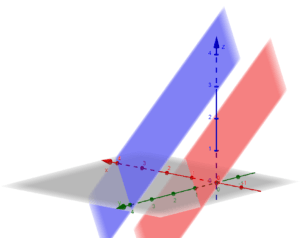
The distance between parallel planes is a simple concept of which we are going to write its formula and we will see an example.
Formula for distance between parallel planes
Vamos a redactar las ecuaciones de los siguientes planos paralelos para poder redactar la fórmula: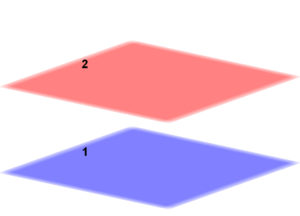
The peculiarity that parallel planes have is that in the coefficients of the unknowns x, y and z they will have the same value and the only thing that is different is the value of the independent term, let’s see at the equation of the plane 1 and plane 2 respectively:
ax + by + cz = d_{1}
ax + by + cz = d_{2}
Once we have understood that the independent term is the only thing that changes, we are going to show the formula to calculate the distance of the parallel planes:
D = \cfrac{\left| d_{2} - d_{1}\right|}{\sqrt{a^{2} + b^{2} + c^{2}}}
Example of distance between parallel planes
Calculate the distance between the planes:
(1) \ \ x + y + z = 4
(2) \ \ 2x + 2y + 2z = 6
As you can see, the coefficients of the unknowns do not have the same values, so to solve this we can multiply equation 1 by 2 or we can divide equation 2 by 2. What we will do is divide equation 2 by 2 to do calculations quickly:
(1) \ \ x + y + z = 4 \quad \quad \quad (2) \ \ x + y + z = 3
Once they already have the same coefficients, we can apply the formula:
D = \cfrac{\left| d_{2} - d_{1}\right|}{\sqrt{a^{2} + b^{2} + c^{2}}} = \cfrac{\left| 3-4\right|}{\sqrt{1^{2} + 1^{2} + 1^{2}}}
D = \cfrac{1}{\sqrt{3}} = \cfrac{\sqrt{3}}{3}
So the distance between parallel planes 1 and 2 is:
D = \cfrac{\sqrt{3}}{3}
Thank you for being in this moment with us : )
