Bisectrix

The definition of bisectrix

It is called bisectrix from an angle to the line, ray or segment that divides this angle into two equal angles. The bisectrixes of the angles of a polygon that are cut at the same point is called incenter. All triangles have an incenter and not all polygons such as quadrilaterals, pentagons, hexagons, etc. have an incenter.

How to draw a bisectrix

Before continuing with the examples, I want to teach how to draw a bisectrix, you just need a compass. We will draw the bisectrix of \measuredangle XYZ.

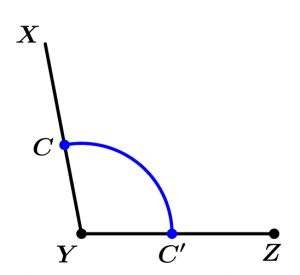
Next we will draw an arc of circumference where the point Y will be the center and the radius can be of the length that you like, thus we will obtain two intersections to which we will name C and C':

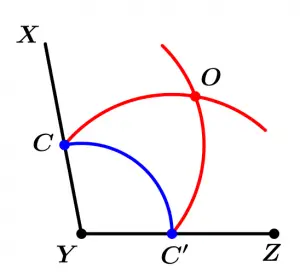
Then we will draw two more circumference arcs, which will have as centers C and C' and radius will be the segment \overline{C C'} and its intersection will be named O:

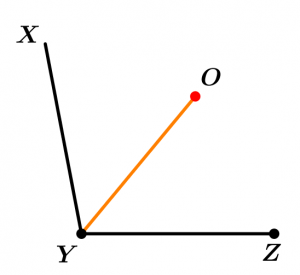
Finally, the segment, ray or line that is plotted with the points Y and O is the bisectrix.

Bisectrix of the \measuredangle XYZ

Graphically it is like this:

Let’s go with the examples of bisectrix

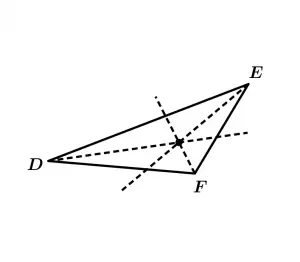
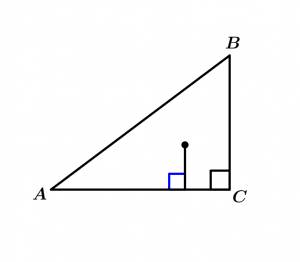
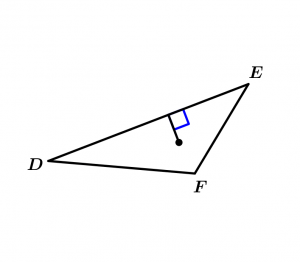
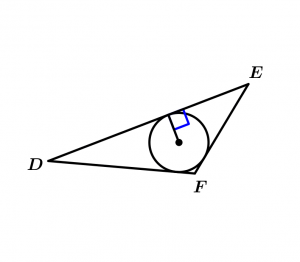
We will calculate the incenter of a triangle, to do this we must calculate the bisectrix of each angle of the triangle. We will work with a right triangle and with a scalene triangle.

\measuredangle ACB = 90^{\text{o}}

\overline{DE} \neq \overline{EF} \neq \overline{FD}

Now let’s draw the bisectors of each of the triangles:

Then we draw a segment that is perpendicular on any of the sides of the triangle, this segment will be the radius of our circumference:

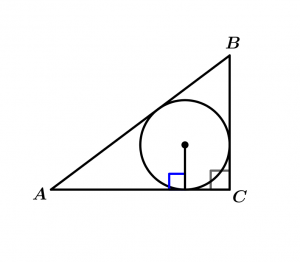
Now we simply draw our inscribed circle:

That’s it, you know how to plot the incenter of any triangle.

Remember: if a polygon has an incenter, it has an inscribed circumference; if a polygon has an inscribed circle, it has an incenter.

Some more examples

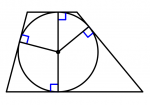
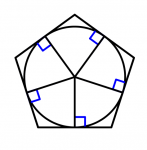
First I want to emphasize that ALL regular polygons have an incenter.

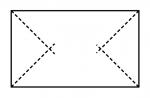
Next we will see some quadrilaterals. You will see that there are irregular quadrilaterals that have an incenter and others that do not. The dotted lines of the quadrilateral without incenter are the bisectors.

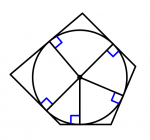
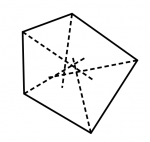
Next we will see some pentagons. You will see that there are irregular pentagons that have an incenter and others that do not. The dotted lines of the pentagon without incenter are the bisectors.

And as you may have noticed, all regular polygons have an incenter and there are irregular polygons that have and do not have an incenter.

You are ready to go out into the world and calculate bisectrixes and incenters!

Thank you for being at this moment with us : )