▷ KIRCHHOFF'S LAW for electric circuits

Kirchhoff’s Voltage Law and Current Law apply to all electrical circuits regardless of whether they are in series, parallel, or series-parallel.

Let us know the following two concepts before starting to solve an electrical circuit:

Mesh

Any closed path in an electrical circuit

Node

The sum of the currents entering or leaving a node is equal to zero.

How to solve an electrical circuit?

Simple and simply they will be solved with Kirchhoff’s Laws:

Kirchhoff’s first Law of the mesh or Kirchhoff’s Voltage Law:

The law says: “The sum of potential drops in a mesh is equal to zero”

Kirchhoff’s second Law of nodes or Kirchhoff’s Current Law:

The law says: “The sum of the currents entering or leaving a node is equal to zero”

Let’s see the following example of electrical circuit circuit

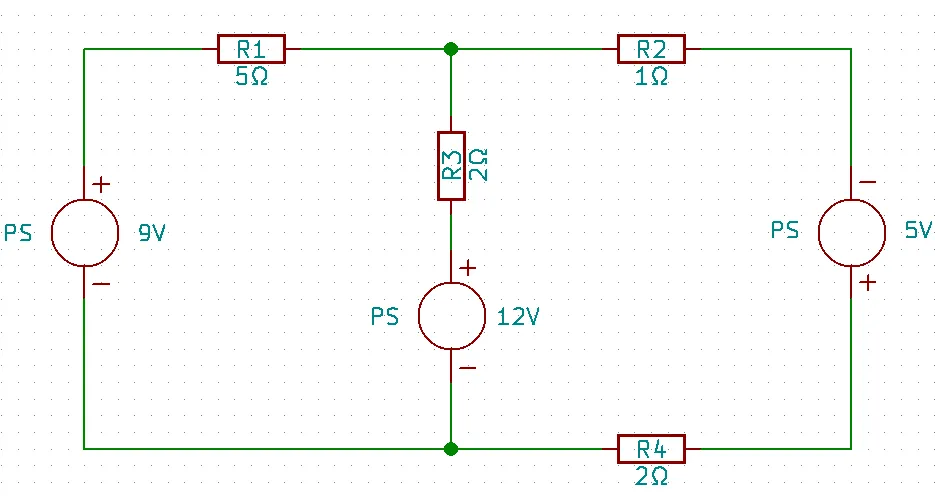
1. Let’s assign all the arbitrary directions in which the current can go from one point to another, in this case as there are only two nodes it would be from node A to node B (see next image), so we can realize that the circuit has 3 intensities, which are represented with Blue ( $I_1$ ), Violet ( $I_2$ ), and Red ( $I_3$ ). Depending on the direction assigned to the arbitrary currents, the resistors will have an arbitrary potential difference from + to – as shown in the following image:

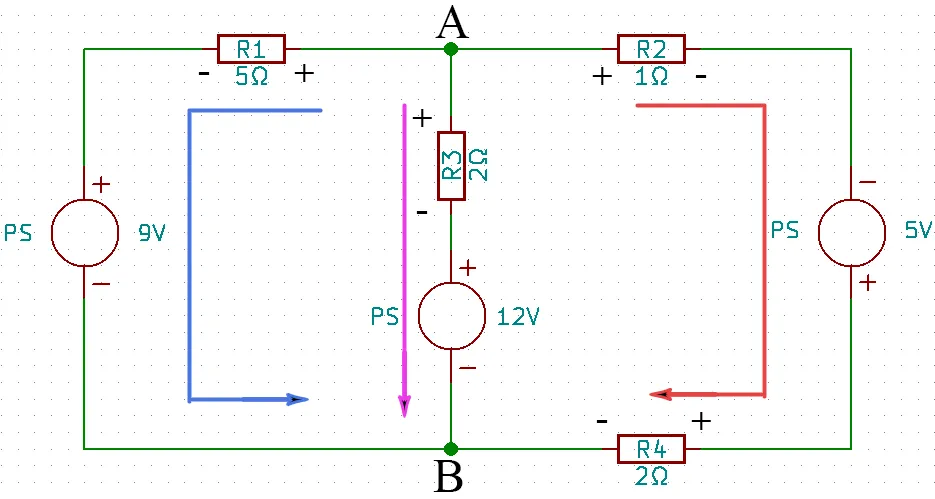
2. With the potential differences placed arbitrarily, we will now place two points on the two meshes to then apply Kirchhoff’s laws:

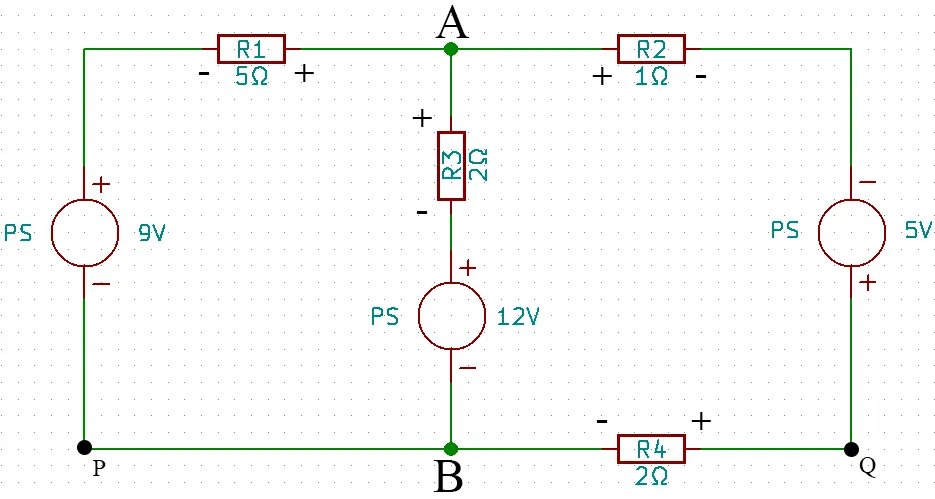
3. We remove the arbitrary intensity lines. Applying the Kirchhoff Law of meshes or Kirchhoff’s voltage law, we have that the sum of the potential drops at point P is equal to zero and in the same way the sum of the potential drops at point Q is equal to zero. Let’s say we’re going to add the potential drops from point P clockwise and those from point Q counterclockwise.

Once we have everything identified, this is where Ohm’s Law and Kirchhoff’s Law come together. Let’s start with point P explaining each drop and/or rise in potential:

The first thing you notice is that there is a rise in potential due to the $9V$ voltage going across the source from the negative sign to the positive sign.
Then another rise in potential occurs as it passes through R1, and we are going to represent that rise in potential as $+5I_{1}$ since the Ohm’s Law formula says that $V = I \times R$ (Voltage equals Current multiplied by Resistance), so the voltage across R1 equals $+5I_{1}$ .
Then what will be observed is that we are going to cross R2 and what will happen is that there will be a drop in potential since it crosses from positive to negative due to the arbitrary signs assigned to it at the beginning, therefore, that drop of potential we will represent it as $-2I_{2}$ .
When crossing the next voltage source of $12V$ , we will observe that there will be a power drop of $12V$
After passing through the $12V$ voltage source, we return to point P. Therefore, the addition and/or subtraction of potential drops would be represented as follows:

$9V + 5I_{1} \ - \ 2I_{2} \ - \ 12V = 0$

We leave the voltages equal to the currents and we already have our first equation:

$5I_{1} \ - \ 2I_{2} \ = 3V$

Let’s continue with point Q explaining each drop and/or rise in potential:

The first thing that happens is that we cross the voltage source of $5V$ observing that it is a potential drop, therefore we will represent it as $-5V$ .
Now we will go through a potential rise in R2, we will represent it as $+I_{3}$ .
There will then be a potential drop across R3, represented as $-2I_{2}$ .
Across the $12V$ voltage source, there will be a potential drop, represented as $-12V$ .
There will now be a potential rise across R4, represented as $+2I_{3}$ .

Adding all the determined terms, we have:

$-5V \ + \ I_{3} \ - \ 2I_{2} \ - \ 12V \ + \ 2I_{3} \ = 0$

Reducing terms and leaving the voltages on one side we have our second equation:

$-2I_{2} \ + \ 3 I_{3} \ = \ 17V$

Now using Kirchhoff’s Law of nodes, we have that the sum of the intensities that leave and enter a node is equal to zero, the node $A$ was used and it was arbitrarily set that all the intensities leave from the node $A$ , and when the intensities leave a node they are subtracted and when they enter a node they are added, therefore our equation would be as follows:

$-I_{1} \ - \ I_{2} \ - \ I_{3} \ = 0$

And once with our 3 equations already defined, we proceed to solve them by the method we want, or if we want we can use a calculator:

$5I_{1} \ - \ 2I_{2} \ + 0 = 3V$

$0 \ - \ 2I_{2} \ + \ 3I_{3} \ = 17V$

$-I_{1} \ - \ I_{2} \ - \ I_{3} = 0$

Where the value of the three intensities is as follows:

$I_{1} = \ - \frac{19}{31}A \approx \ -0.6129 A$

$I_{2} = \ - \frac{94}{31}A \approx \ -3.0322 A$

$I_{3} = \frac{113}{31} A \approx 3.6451 A$

What does it mean to have negative intensities?

All it means is that the current is not actually going in the arbitrary direction we told at the beginning, but rather in the opposite direction, which means that $I_{1}$ and $I_{2}$ have to go in the opposite direction, in addition to the signs of the potential drops that we assign the same would have to change with respect to the direction of the current, which results in the final scheme being as follows way:

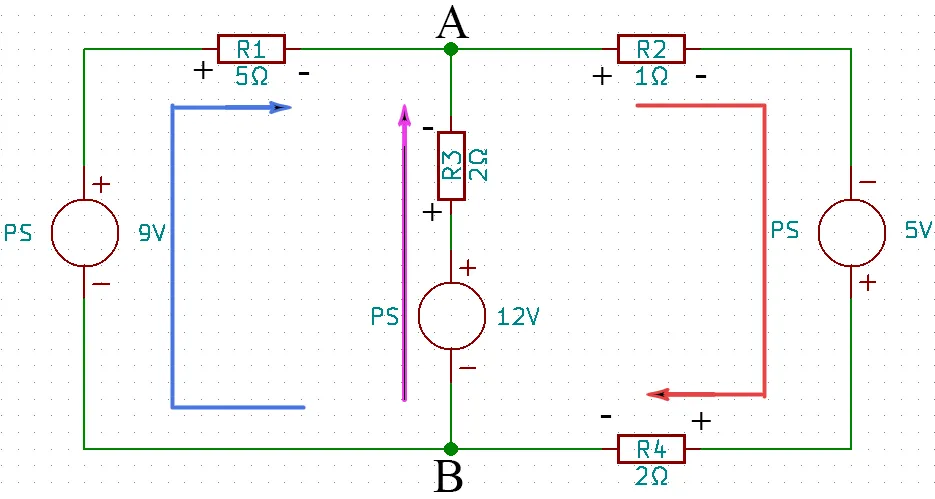
Then the current intensities would be as follows:

$I_{1} = \frac{19}{31}A \approx 0.6129 A$

$I_{2} = \frac{94}{31}A \approx 3.0322 A$

$I_{3} = \frac{113}{31} A \approx 3.6451 A$

And with the intensities found you can determine the potential drop of each resistor!

Thank you for being in this moment with us : )